Promotion
Use code MOM24 for 20% off site wide + free shipping over $45
Math Games with Bad Drawings
75 1/4 Simple, Challenging, Go-Anywhere Games—And Why They Matter
Contributors
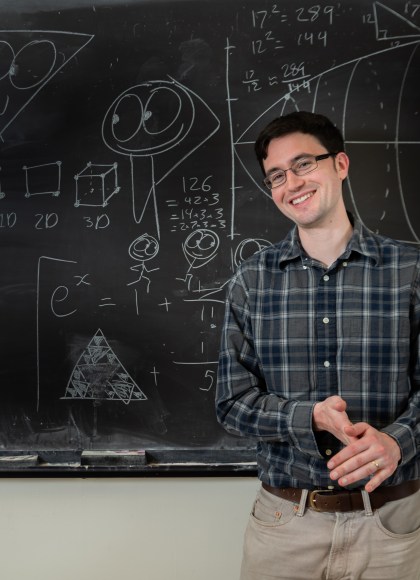
By Ben Orlin
Formats and Prices
Price
$30.00Price
$39.00 CADFormat
Format:
- Hardcover $30.00 $39.00 CAD
- Kit $16.99 $22.99 CAD
- ebook $15.99 $20.99 CAD
This item is a preorder. Your payment method will be charged immediately, and the product is expected to ship on or around April 5, 2022. This date is subject to change due to shipping delays beyond our control.
Also available from:
Bestselling author and worst-drawing artist Ben Orlin expands his oeuvre with this interactive collection of mathematical games. With 70-plus games, each taking a minute to learn and a lifetime to master, this treasure trove will delight, educate, and entertain.
From beloved math popularizer Ben Orlin comes a masterfully compiled collection of dozens of playable mathematical games.This ultimate game chest draws on mathematical curios, childhood classics, and soon-to-be classics, each hand-chosen to be (1) fun, (2) thought-provoking, and (3) easy to play. With just paper, pens, and the occasional handful of coins, you and a partner can enjoy hours of fun—and hours of challenge.
Orlin’s sly humor, expansive knowledge, and so-bad-they’re-good drawings show us how simple rules summon our best thinking.
Games include:
- Ultimate Tic-Tac-Toe
- Sprouts
- Battleship
- Quantum Go Fish
- Dots and Boxes
- Black Hole
- Order and Chaos
- Sequencium
- Paper Boxing
- Prophecies
- Arpeggios
- Banker
- Francoprussian Labyrinth
- Cats and Dogs
- And many more.
Genre:
-
“I’m loving this math book with puzzles. Such a gentle, playful way to teach these abstract concepts. Like a pill pocket for math!”Allie Brosh, author of Hyperbole and a Half
-
“Ben Orlin is such an interesting thinker, he is a wonderful mix of informed, funny and creative. This book is a delight, pages and pages of engaging math games. I will carry this with me everywhere, and my mathematical thinking will be better because of it.”Jo Boaler, Stanford Professor & cofounder of youcubed
-
"Mathematicians keep insisting that math is fun, but most people who have taken a math class might be skeptical. Ben Orlin's new book of mathematical games reveals where the fun has been lurking all along. The games themselves are easy to learn, promise hours of enjoyment, and will introduce you to some pretty advanced concepts along the way."Sean Carroll, author of Something Deeply Hidden: Quantum Worlds and the Emergence of Spacetime
-
"A beautifully produced compendium of maths games that is destined to be a classic of popular maths literature."Alex Bellos, The Guardian
-
"So good, I'm not even jealous.... It's just stellar."Dan Finkel, co-creator of the award-winning games Prime Climb and Tiny Polka Dot
- On Sale
- Apr 5, 2022
- Page Count
- 368 pages
- Publisher
- Black Dog & Leventhal
- ISBN-13
- 9780762499861
Newsletter Signup
By clicking ‘Sign Up,’ I acknowledge that I have read and agree to Hachette Book Group’s Privacy Policy and Terms of Use